If you are interested in trying to understand physics, either at a technical level or at an amateur level, at some point you will inevitably hear a physicist waxing on about the beauty of the physical laws. This might sound very strange if your view of physics is tedious calculations and horrible professors tricking you on multiple choice questions. So, what then do physicists mean when they talk about the beauty of the laws of physics? In my experience (talking to physicists and studying the laws ), what they are generally referring to is the delicate balance between the rigidity and fragility of the laws of physics, that is to say, the realization that the whole structure rests on a few principles, any of which removed would bring the whole structure tumbling down. As an example that is not at all obvious, suppose you disobey the principles of special relativity, then you necessarily violate causality. The source of this property (rigidity and fragility) is the idea of symmetry.
We are all intuitively familiar with the idea of symmetry and easily recognize it in photography and paintings that move us. What is largely under-appreciated is how much of these concepts of symmetry are applicable to the laws of physics and, more importantly, how they expand on what we mean by symmetry. For the purposes of this discussion, what we mean by symmetry is any operation that can be done to an object and also, astoundingly, an equation that leaves the object or the equation as it was before. Put another way, it is equivalent to doing nothing or applying the identity operation. Take for example, rotation of a square by 90 degrees. This leaves the square looking as it was before; the operation is the rotation and the object is the square. The same logic applies for the operation being a mathematical operation and the object being an equation. An mathematical operation means something I can do to the equation. For example adding a number to the equation is an example of an operation. I shall distinguish between physical and abstract symmetries in the discussion that follows.
Physical Symmetries
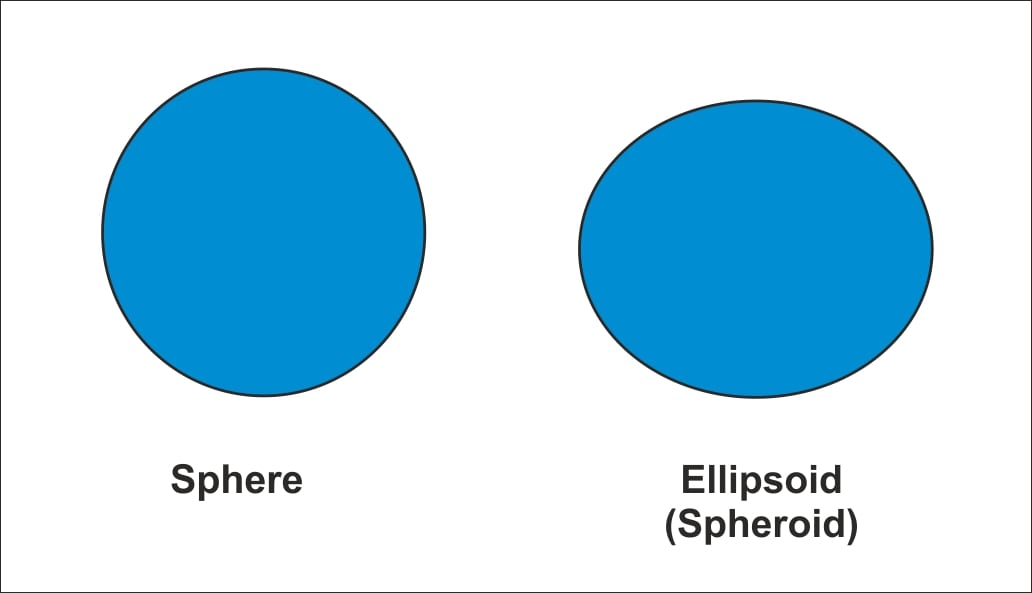
These are the symmetries that are the most familiar. Consider a sphere, a rotation by any angle about any axis leaves the sphere invariant (the sphere looks as though you had done nothing to it). On the other hand think of an ellipsoid; there are clearly distinguished directions and angles that rotations will keep the ellipsoid invariant, and others that will not.
Left: Ellipsoid : Rotating about the elongated axis. Right: Sphere: Rotating about any axis leaves the picture the same leaves the picture the same. Image Credit: Bayes Ahmed via Flickr.
So how might such rotational symmetry appear in the laws of physics? Suppose you put a hydrogen atom in a spherically symmetric environment, i.e. in an ambient space that looks the same in all directions. It turns out there are physical consequences to doing this. Now one can solve the equations for the system and find out all the possible energies the system can have. Merely consider one of them and ask yourself how many quantum states (a physical state a quantum system can be in) that the system can be in that will have this energy. It turns out that as a consequence of the spherical symmetry there will be more than one state with the same energy. Physicists call this phenomenon degeneracy. However, we may break the symmetry by putting a magnetic field pointing in some direction (like putting a magnet near by). This means the magnetic field picks out a distinguished position (distinguished by the fact that the magnetic field points in that very direction and no other). What then occurs? All the quantum states that had the same energy as a consequence of the symmetry suddenly have different energies.
As another example, look at the picture below.
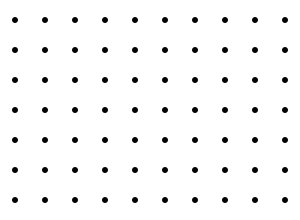
There is a certain regularity to the lattice. If we suppose the distance between the dots is distance a, then moving a to the north, south, west and east means that the world on this lattice looks the same. Let's suppose that these dots represent electrons; then as a consequence of this lattice symmetry, the nature of the wave function (quantum state) of the atoms is completely determined (this rarely happens) This is important because the wave function contains all the information about the quantum system that you are allowed to know. The lattice has a translational symmetry that can be used to determine what quantum state the atoms can be in.
Abstract Symmetries: Emmy's World
Abstract symmetries occur where the operation is done on the equation and the operation is not necessarily something that is physically realizable.The first person to study such symmetries in a formal manner was the female mathematician, Emmy Noether (one of the most brilliant mathematicians of the past century who greatly influenced the direction of twentieth century physics). She proved what I consider to be the most astounding result of mathematical physics in the last hundred years:
To every continuous symmetry there is a conserved quantity
Let's unpack the statement. What does continuous mean? Let's suppose your equation has the variable x and that you can imagine changing x to x + dx, where dx simply means an infinitesimal change of x which is added to x . Infinitesimal means we can make the change so small that there would no way to measure it. The fact that we can make the change infinitesimal means our variable is continuous and furthermore if this change is some symmetry operation (mathematical operation that leaves the equations the same) then we have continuous symmetry; the operation continuously changes our equations but leaves as they were after it is done. But as a consequence of this, Emmy proved there is some quantity that does not change in time; it is conserved. What quantity we refer to will depend on the symmetry and sometimes the system.
Say, we have an equation that has the variable that represents time. Now, as far as we know time is a continuous parameter. If our equations are invariant under an operation that changes time then according to Emmy there is a conserved quantity. What is that quantity? It is the total energy of the system. We may put this more physically; suppose that my equations do not care whether I do the experiment in the year 1729 or 2067. Then according to Noether, as a consequence of this, we must conserve the total energy, meaning the total energy stays the same as long this fact is true. Or we could consider another kind of symmetry. Assume your equations do not care at what point in space you do the experiment, this necessarily results in conservation of total linear momentum. You could ask why knowing these conserved quantities matters? The answer is that it usually makes understanding the dynamics of the system a lot easier. The more conserved quantities there are, the simpler the equations can be made to look and solve.
Noether used the powerful language of group theory to formulate her theorem. Simply put, a group is a mathematical structure that encodes in a convenient fashion what symmetry one is considering. This enabled physicists to find extremely abstract symmetries that governed the laws of nature. For example, in some particle reactions physicists noted that it did not matter whether you have a proton or neutron. Put another way, the equations did not care whether you started with a proton or a neutron. So there must be some abstract symmetry that the equations have. Heisenberg introduced the symmetry of a 3 dimensional hypersphere to turn a proton into a neutron and vice versa. So there is some sense in which the proton and the neutron are the same, i.e there is a symmetry operation in the equations that turns one into the other.
Symmetries of physics are the only example I know where Keats' exhortation is literally true
When old age shall this generation waste,
Thou shalt remain, in midst of other woe
Than ours, a friend to man, to whom thou sayst,
“Beauty is truth, truth beauty,” – that is all
Ye know on earth, and all ye need to know
It is a most incredible fact that the desire for beauty in so far as symmetry is sought is not a mere bootless cry; in physics the beauty can essentially tell you the truth. Can you think of an endeavour in life where the search of beauty is synonymous with the search of truth? This is the beauty that captures all those who study and comprehend it.
About the author:
 |
Amara Katabarwa is a PhD candidate in the Physics and Astronomy Department at the University of Georgia studying His research focus is understanding Decoherence in Quantum Circuits and near term application of first generation Quantum Computers. In his free time he likes to read or guiltlessly laze about. He can be contacted at akataba@uga.edu. |
